Glmnet: Difference between revisions

From 太極
Jump to navigation Jump to search
Line 169: Line 169:
| BhGLM
| BhGLM
| measure.bh(fit, new.x, new.y)
| measure.bh(fit, new.x, new.y)
| cv.bh(fit, nfolds=10, foldid, ncv)$measures[1]<br />  which calls an internal function measure.cox(obj, lp)<br />  which calls bcoxph() [coxph()] using lp as the covariate.
| cv.bh(fit, nfolds=10, foldid, ncv)$measures[1]<br />  which calls an internal function measure.cox(obj, lp)<br />  which calls bcoxph() [coxph()] using lp as the covariate<br />and deviance = -2 * ff$loglik[2] <br />cindex = <nowiki>summary(ff)$concordance[[1]]</nowiki>
|}
|}


Revision as of 10:30, 19 June 2020

Basic

  • https://en.wikipedia.org/wiki/Lasso_(statistics). It has a discussion when two covariates are highly correlated. For example if gene [math]\displaystyle{ i }[/math] and gene [math]\displaystyle{ j }[/math] are identical, then the values of [math]\displaystyle{ \beta _{j} }[/math] and [math]\displaystyle{ \beta _{k} }[/math] that minimize the lasso objective function are not uniquely determined. Elastic Net has been designed to address this shortcoming.
    • Strongly correlated covariates have similar regression coefficients, is referred to as the grouping effect. From the wikipedia page "one would like to find all the associated covariates, rather than selecting only one from each set of strongly correlated covariates, as lasso often does. In addition, selecting only a single covariate from each group will typically result in increased prediction error, since the model is less robust (which is why ridge regression often outperforms lasso)".
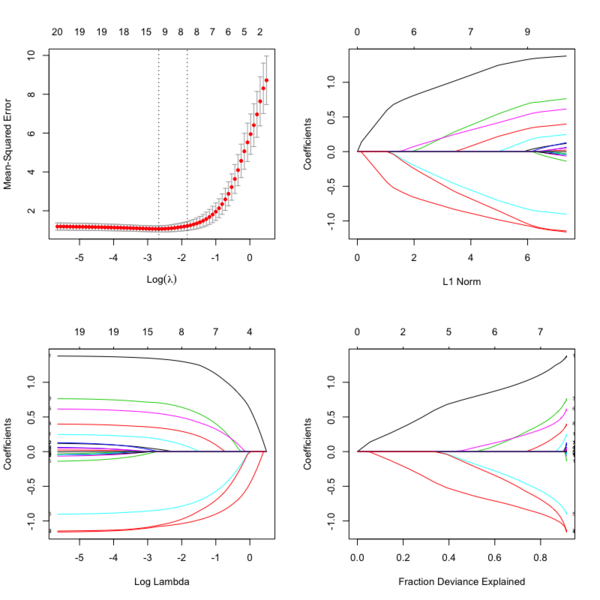
  • Glmnet Vignette. It tries to minimize [math]\displaystyle{ RSS(\beta) + \lambda [(1-\alpha)\|\beta\|_2^2/2 + \alpha \|\beta\|_1] }[/math]. The elastic-net penalty is controlled by [math]\displaystyle{ \alpha }[/math], and bridge the gap between lasso ([math]\displaystyle{ \alpha = 1 }[/math]) and ridge ([math]\displaystyle{ \alpha = 0 }[/math]). Following is a CV curve (adaptive lasso) using the example from glmnet(). Two vertical lines are indicated: left one is lambda.min (that gives minimum mean cross-validated error) and right one is lambda.1se (the most regularized model such that error is within one standard error of the minimum). For the tuning parameter [math]\displaystyle{ \lambda }[/math],
    • The larger the [math]\displaystyle{ \lambda }[/math], more coefficients are becoming zeros (think about coefficient path plots) and thus the simpler (more regularized) the model.
    • If [math]\displaystyle{ \lambda }[/math] becomes zero, it reduces to the regular regression and if [math]\displaystyle{ \lambda }[/math] becomes infinity, the coefficients become zeros.
    • In terms of the bias-variance tradeoff, the larger the [math]\displaystyle{ \lambda }[/math], the higher the bias and the lower the variance of the coefficient estimators.

File:Glmnetplot.svg File:Glmnet path.svg File:Glmnet l1norm.svg 

set.seed(1010)
n=1000;p=100
nzc=trunc(p/10)
x=matrix(rnorm(n*p),n,p)
beta=rnorm(nzc)
fx= x[,seq(nzc)] %*% beta
eps=rnorm(n)*5
y=drop(fx+eps)
px=exp(fx)
px=px/(1+px)
ly=rbinom(n=length(px),prob=px,size=1)

## Full lasso
set.seed(999)
cv.full <- cv.glmnet(x, ly, family='binomial', alpha=1, parallel=TRUE)
plot(cv.full)  # cross-validation curve and two lambda's
plot(glmnet(x, ly, family='binomial', alpha=1), xvar="lambda", label=TRUE) # coefficient path plot
plot(glmnet(x, ly, family='binomial', alpha=1))  # L1 norm plot
log(cv.full$lambda.min) # -4.546394
log(cv.full$lambda.1se) # -3.61605
sum(coef(cv.full, s=cv.full$lambda.min) != 0) # 44

## Ridge Regression to create the Adaptive Weights Vector
set.seed(999)
cv.ridge <- cv.glmnet(x, ly, family='binomial', alpha=0, parallel=TRUE)
wt <- 1/abs(matrix(coef(cv.ridge, s=cv.ridge$lambda.min)
                   [, 1][2:(ncol(x)+1)] ))^1 ## Using gamma = 1, exclude intercept
## Adaptive Lasso using the 'penalty.factor' argument
set.seed(999)
cv.lasso <- cv.glmnet(x, ly, family='binomial', alpha=1, parallel=TRUE, penalty.factor=wt)
# defautl type.measure="deviance" for logistic regression
plot(cv.lasso)
log(cv.lasso$lambda.min) # -2.995375
log(cv.lasso$lambda.1se) # -0.7625655
sum(coef(cv.lasso, s=cv.lasso$lambda.min) != 0) # 34

Lambda [math]\displaystyle{ \lambda }[/math]

Mixing parameter [math]\displaystyle{ \alpha }[/math]

cva.glmnet() from the glmnetUtils package to choose both the alpha and lambda parameters via cross-validation, following the approach described in the help page for cv.glmnet.

Underfittig, overfitting and relaxed lasso

Plots

library(glmnet)
data(QuickStartExample) # x is 100 x 20 matrix

cvfit = cv.glmnet(x, y)
fit = glmnet(x, y)

oldpar <- par(mfrow=c(2,2))
plot(cvfit) # mse vs log(lambda)
plot(fit) # coef vs L1 norm
plot(fit, xvar = "lambda", label = TRUE) # coef vs log(lambda)
plot(fit, xvar = "dev", label = TRUE) # coef vs Fraction Deviance Explained
par(oldpar)

Glmnet4plotn.png

print() method

?print.glmnet and ?print.cv.glmnet

Extract/compute deviance

deviance(fitted_glmnet_object)

coxnet.deviance(pred = NULL, y, x = 0, offset = NULL,
  weights = NULL, beta = NULL)

cv.glmnet and deviance

Usage 

cv.glmnet(x, y, weights = NULL, offset = NULL, lambda = NULL,
  type.measure = c("default", "mse", "deviance", "class", "auc", "mae",
  "C"), nfolds = 10, foldid = NULL, alignment = c("lambda",
  "fraction"), grouped = TRUE, keep = FALSE, parallel = FALSE,
  gamma = c(0, 0.25, 0.5, 0.75, 1), relax = FALSE, trace.it = 0, ...)

type.measure parameter (loss to use for CV):

  • default = deviance which uses squared-error for gaussian models (a.k.a type.measure="mse"), logistic and poisson regresion, and partial-likelihood for the Cox model. PS: for binary response data I found that type='class' gives a discontinuous CV curve while 'deviance' give a smooth CV curve.
  • mse or mae (mean absolute error) can be used by all models except the "cox"; they measure the deviation from the fitted mean to the response
  • class applies to binomial and multinomial logistic regression only, and gives misclassification error.
  • auc is for two-class logistic regression only, and gives area under the ROC curve.
  • C is Harrel's concordance measure, only available for cox models
package deviance CV
survival -2*fit$loglik[1]
glmnet deviance(fit)
assess.glmnet(fit, newx, newy, family = "cox") 		cv.glmnet(x, y, lambda, family="cox", nfolds, foldid)$cvm
BhGLM measure.bh(fit, new.x, new.y) cv.bh(fit, nfolds=10, foldid, ncv)$measures[1]
which calls an internal function measure.cox(obj, lp)
which calls bcoxph() [coxph()] using lp as the covariate
and deviance = -2 * ff$loglik[2]
cindex = summary(ff)$concordance[[1]]

No need for glmnet if we have run cv.glmnet

https://stats.stackexchange.com/a/77549 Do not supply a single value for lambda. Supply instead a decreasing sequence of lambda values. glmnet relies on its warms starts for speed, and its often faster to fit a whole path than compute a single fit.

cv.glmnet in cox model

Note that the y-axis on the plot depends on the type.measure parameter. It is not the objective function used to find the estimator. For survival data, the y-axis is deviance (-2*loglikelihood) [so the optimal lambda should give a minimal deviance value].

It is not always partial likelihood device has a largest value at a large lambda. In the following two plots, the first one is from the glmnet vignette and the 2nd one is from the coxnet vignette. The survival data are not sparse in both examples.

Cvcoxnet.png

Sparse data 

library(glmnet); library(survival)
n = 100; p <- 1000
beta1 = 2; beta2 = -1; beta3 =1; beta4 = -2
lambdaT = .002 # baseline hazard
lambdaC = .004  # hazard of censoring
set.seed(1234)
x1 = rnorm(n)
x2 = rnorm(n)
x3 <- rnorm(n)
x4 <- rnorm(n)
# true event time
T = Vectorize(rweibull)(n=1, shape=1, scale=lambdaT*exp(-beta1*x1-beta2*x2-beta3*x3-beta4*x4))
C = rweibull(n, shape=1, scale=lambdaC)   #censoring time
time = pmin(T,C)  #observed time is min of censored and true
event = time==T   # set to 1 if event is observed
cox <- coxph(Surv(time, event)~ x1 + x2 + x3 + x4); cox
-2*cox$loglik[2] # deviance [1] 301.7461
summary(cox)$concordance[1]  # 0.9006085

# create a sparse matrix
X <- cbind(x1, x2, x3, x4, matrix(rnorm(n*(p-4)), nr=n))
colnames(X) <- paste0("x", 1:p)
# X <- data.frame(X)
y <- Surv(time, event)
set.seed(1234)
nfold <- 10
foldid <- sample(rep(seq(nfold), length = n))
cvfit <- cv.glmnet(X, y, family = "cox", foldid = foldid)
plot(cvfit)
assess.glmnet(cvfit, newx=X, newy = y, family="cox") # return deviance 361.4619 and C 0.897421
            # Question: what lambda has been used?
            # Ans: assess.glmnet() calls predict.cv.glmnet() which by default uses s = "lambda.1se"

fit <- glmnet(X, y, family = "cox", lambda = cvfit$lambda.min)
assess.glmnet(fit, newx=X, newy = y, family="cox") # deviance 308.3646 and C 0.9382788

fit <- glmnet(X, y, family = "cox", lambda = cvfit$lambda.1se)
assess.glmnet(fit, newx=X, newy = y, family="cox") # deviance  361.4786 and C 0.897421
deviance(fit)
# [1] 361.4786

library(BhGLM)
X2 <- data.frame(X)
f1 = bmlasso(X2, y, family = "cox",  ss = c(.04, .5))
measure.bh(f1, X2, y)
# deviance   Cindex
#   303.39     0.90

o <- cv.bh(f1, foldid = foldid)
o$measures  # deviance and C
# deviance   Cindex
#  311.743    0.895

update() function

update() will update and (by default) re-fit a model. It does this by extracting the call stored in the object, updating the call and (by default) evaluating that call. Sometimes it is useful to call update with only one argument, for example if the data frame has been corrected.

It can be used in glmnet() object without a new implementation method.

  • Linear regression 
    lm(y ~ x + z, data=myData)
lm(y ~ x + z, data=subset(myData, sex=="female"))
lm(y ~ x + z, data=subset(myData, age > 30))
  • Lasso regression 
    R> fit <- glmnet(glmnet(X, y, family="cox", lambda=cvfit$lambda.min); fit
Call:  glmnet(x = X, y = y, family = "cox", lambda = cvfit$lambda.min)

  Df   %Dev Lambda
1 21 0.3002 0.1137

R> fit2 <- update(fit, subset = c(rep(T, 50), rep(F, 50)); fit2
Call:  glmnet(x = X[1:50, ], y = y[1:50], family = "cox", lambda = cvfit$lambda.min)

  Df   %Dev Lambda
1 24 0.4449 0.1137

R> fit3 <- update(fit, lambda=cvfit$lambda); fit3

Call:  glmnet(x = X, y = y, family = "cox", lambda = cvfit$lambda)

    Df    %Dev  Lambda
1    1 0.00000 0.34710
2    2 0.01597 0.33130
...

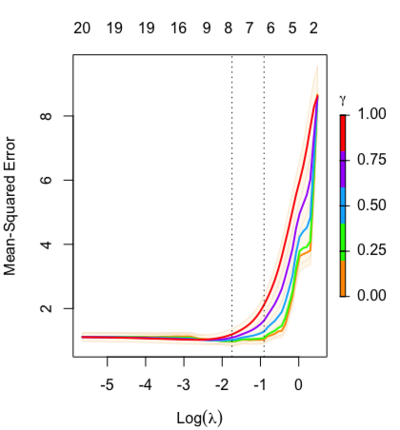
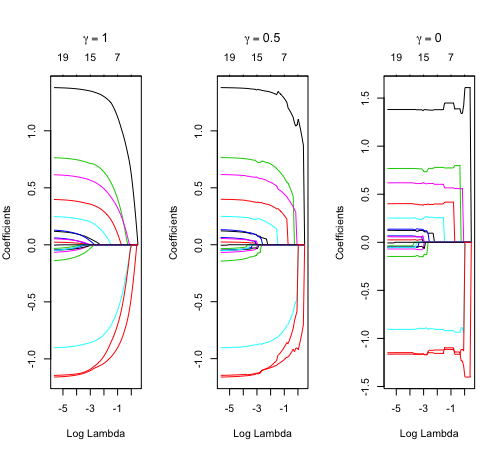
Relaxed fit and [math]\displaystyle{ \gamma }[/math] parameter

https://cran.r-project.org/web/packages/glmnet/vignettes/relax.pdf

Relaxed fit: Take a glmnet fitted object, and then for each lambda, refit the variables in the active set without any penalization.

Suppose the glmnet fitted linear predictor at [math]\displaystyle{ \lambda }[/math] is [math]\displaystyle{ \hat\eta_\lambda(x) }[/math] and the relaxed version is [math]\displaystyle{ \tilde\eta_\lambda(x) }[/math]. We also allow for shrinkage between the two:

[math]\displaystyle{ \begin{align} \tilde \eta_{\lambda,\gamma}= \gamma\hat\eta_\lambda(x) + (1-\gamma)\tilde\eta_\lambda(x). \end{align} }[/math]

[math]\displaystyle{ \gamma\in[0,1] }[/math] is an additional tuning parameter which can be selected by cross validation.

The debiasing will potentially improve prediction performance, and CV will typically select a model with a smaller number of variables.

The default behavior of extractor functions like predict and coef, as well as plot will be to present results from the glmnet fit (not cv.glmnet), unless a value of [math]\displaystyle{ \gamma }[/math] is given different from the default value [math]\displaystyle{ \gamma=1 }[/math].

Question: how does cv.glmnet() select [math]\displaystyle{ \gamma }[/math] parameter? Ans: it depends on the parameter type.measure in cv.glmnet. 

library(glmnet)
data(QuickStartExample)
fitr=glmnet(x,y, relax=TRUE)
set.seed(1)
cfitr=cv.glmnet(x,y,relax=TRUE)
c(fitr$lambda.min, fitr$lambda.1se)
# [1] 0.08307327 0.15932708
str(cfitr$relaxed)
plot(cfitr),oldpar <- par(mfrow=c(1,3), mar = c(5,4,6,2))
plot(fitr, main = expression(gamma == 1))
plot(fitr,gamma=0.5, main = expression(gamma == .5))
plot(fitr,gamma=0, main = expression(gamma == 0))
par(oldpar)
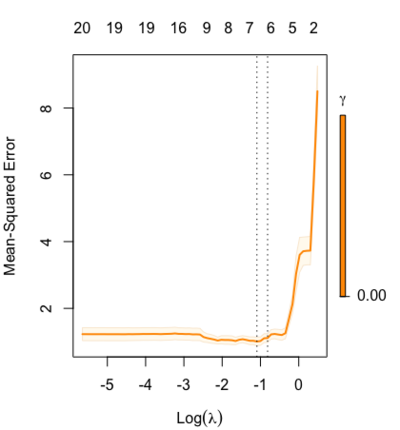
Special cases:
  • [math]\displaystyle{ \gamma=1 }[/math]: only regularized fit, no relaxed fit.
  • [math]\displaystyle{ \gamma=0 }[/math]: only relaxed fit; a faster version of forward stepwise regression.
set.seed(1)
cfitr2=cv.glmnet(x,y,gamma=0,relax=TRUE) 
  # default gamma = c(0, 0.25, 0.5, 0.75, 1)
plot(cfitr2)
c(cfitr2$lambda.min, cfitr2$lambda.1se)
# [1] 0.08307327 0.15932708
str(cfitr2$relaxed)

Glmnetrelaxcv.png Glmnetrelaxfit.png

Glmnetrelaxcv2.png

Computation time

beta1 = 2; beta2 = -1
lambdaT = .002 # baseline hazard
lambdaC = .004  # hazard of censoring
set.seed(1234)
x1 = rnorm(n)
x2 = rnorm(n)
# true event time
T = Vectorize(rweibull)(n=1, shape=1, scale=lambdaT*exp(-beta1*x1-beta2*x2)) 
# No censoring
event2 <- rep(1, length(T))

system.time(fit <- cv.glmnet(x, Surv(T,event2), family = 'cox'))
#    user  system elapsed 
#   4.701   0.016   4.721 
system.time(fitr <- cv.glmnet(x, Surv(T,event2), family = 'cox', relax= TRUE))
#    user  system elapsed 
# 161.002   0.382 161.573

predict() and coef() methods

?predict.glmnet OR ?coef.glmnet OR ?coef.relaxed. Similar to other predict methods, this functions predicts fitted values, logits, coefficients and more from a fitted "glmnet" object. 

## S3 method for class 'glmnet'
predict(object, newx, s = NULL, type = c("link",
  "response", "coefficients", "nonzero", "class"), exact = FALSE,
  newoffset, ...)

## S3 method for class 'relaxed'
predict(object, newx, s = NULL, gamma = 1,
  type = c("link", "response", "coefficients", "nonzero", "class"),
  exact = FALSE, newoffset, ...)
## S3 method for class 'glmnet'
coef(object, s = NULL, exact = FALSE, ...)

?predict.cv.glmnet OR ?coef.cv.glmnet OR ?coef.cv.relaxed. This function makes predictions from a cross-validated glmnet model, using the stored "glmnet.fit" object, and the optimal value chosen for lambda (and gamma for a 'relaxed' fit). 

## S3 method for class 'cv.glmnet'
predict(object, newx, s = c("lambda.1se",
  "lambda.min"), ...)

## S3 method for class 'cv.relaxed'
predict(object, newx, s = c("lambda.1se",
  "lambda.min"), gamma = c("gamma.1se", "gamma.min"), ...)

Cindex

https://www.rdocumentation.org/packages/glmnet/versions/3.0-2/topics/Cindex

Usage: 

Cindex(pred, y, weights = rep(1, nrow(y)))

assess.glmnet

https://www.rdocumentation.org/packages/glmnet/versions/3.0-2/topics/assess.glmnet

Usage: 

assess.glmnet(object, newx = NULL, newy, weights = NULL,
  family = c("gaussian", "binomial", "poisson", "multinomial", "cox",
  "mgaussian"), ...)
confusion.glmnet(object, newx = NULL, newy, family = c("binomial",
  "multinomial"), ...)

roc.glmnet(object, newx = NULL, newy, ...)

ridge regression

# example from SGL package
set.seed(1)
n = 50; p = 100; size.groups = 10
X = matrix(rnorm(n * p), ncol = p, nrow = n)
beta = (-2:2)
y = X[,1:5] %*% beta + 0.1*rnorm(n)
data = list(x = X, y = y)

cvfit <- cv.glmnet(X, y, alpha = 0)
plot(cvfit)

o <- coef(cvfit, lambda = cvfit$lambda.min) %>% drop()

sum(o != 0) # [1] 101. 
            # Too biased.
o[1:10] 
#   (Intercept)            V1            V2            V3            V4 
# -3.269401e-01 -2.253226e-36 -8.900799e-37  5.198885e-37  1.311976e-36 
#            V5            V6            V7            V8            V9 
#  1.873125e-36  1.582532e-37  2.085781e-37  4.732839e-37  2.997614e-37

y_predicted <- predict(cvfit, s = cvfit$lambda.min, newx = X)
# Sum of Squares Total and Error
sst <- sum((y - mean(y))^2)
sse <- sum((y_predicted - y)^2)
# R squared
rsq <- 1 - sse / sst
rsq  # 0.46

library(SGL)  # sparse group lasso
set.seed(1)
index <- ceiling(1:p / size.groups)
cvFit = cvSGL(data, index, type = "linear", alpha=.95) # this alpha is the default
plot(cvFit)
cvFit$fit$beta[, 20] # 20th lambda gives smallest negative log likelihood  
                     # identify correct predictors
#  [1] -10.942712  -6.167799   0.000000   6.595406  14.442019   0.000000 ...

set.seed(1)
cvFit2 = cvSGL(data, index, type = "linear", alpha=0)
plot(cvFit2)
cvFit2$fit$beta[, 20]
#  [1] -10.8417371  -6.5251240   0.2476438   6.7223001  14.1605263   0.2149542
#  [7]   0.2481450   0.1404282   0.1799818   0.3784596   0.0000000   0.0000000 ...
  • Tikhonov regularization (ridge regression). It was used to handle ill-posed/overfitting situation. Ridge regression shrinks the coefficients by a uniform factor of [math]\displaystyle{ {\displaystyle (1+N\lambda )^{-1}}{\displaystyle (1+N\lambda )^{-1}} }[/math] and does not set any coefficients to zero.
  • cvSGL
  • How and when: ridge regression with glmnet. On training data, ridge regression fits less well than the OLS but the parameter estimate is more stable. So it does better in prediction because it is less sensitive to extreme variance in the data such as outliers.

Group lasso

  • pcLasso: Principal Components Lasso package
    • pclasso paper, slides, Blog
    • Each feature must be assigned to a group
    • It allows to assign each feature to groups (including overlapping). 
      library(pcLasso)

set.seed(1)
n = 50; p = 100; size.groups = 10
index <- ceiling(1:p / size.groups)
X = matrix(rnorm(n * p), ncol = p, nrow = n)
beta = (-2:2)
y = X[,1:5] %*% beta + 0.1*rnorm(n)

groups <- vector("list", 3)
for (k in 1:2) {
    groups[[k]] <- 5 * (k-1) + 1:5
}
groups[[3]] <- 11:p
cvfit <- cv.pcLasso(X, y, ratio = 0.8, groups = groups)
plot(cvfit)

pred.pclasso <- predict(cvfit, xnew = X, s = "lambda.min")
mean((y-pred.pclasso)^2)  #  [1] 1.956387

library(SGL)
index <- ceiling(1:p / size.groups)
data = list(x = X, y = y)
set.seed(1)
cvFit = cvSGL(data, index, type = "linear")
Fit = SGL(data, index, type = "linear")
  # SGL() uses a different set of lambdas than cvSGL() does
  # After looking at cvFit$lambdas; Fit$lambdas
  # I should pick the last lambda 
pred.SGL <- predictSGL(Fit, X, length(Fit$lambdas))
mean((y-pred.SGL)^2)    # [1] 0.146027

library(ggplot2)
library(tidyr)
dat <- tibble(y=y, SGL=pred.SGL, pclasso=pred.pclasso) %>% gather("method", "predict", 2:3)

ggplot(dat, aes(x=y, y=predict, color=method)) + geom_point(shape=1)

Minimax concave penalty (MCP)

penalty.factor

The is available in glmnet() but not in cv.glmnet().

Adaptive lasso and weights

Oracle property and adaptive lasso

  • Variable Selection via Nonconcave Penalized Likelihood and Its Oracle Properties, Fan & Li (2001) JASA
  • Adaptive Lasso: What it is and how to implement in R. Adaptive lasso weeks to minimize [math]\displaystyle{ RSS(\beta) + \lambda \sum_1^p \hat{\omega}_j |\beta_j| }[/math] where [math]\displaystyle{ \lambda }[/math] is the tuning parameter, [math]\displaystyle{ \hat{\omega}_j = \frac{1}{(|\hat{\beta}_j^{ini}|)^\gamma} }[/math] is the adaptive weights vector and [math]\displaystyle{ \hat{\beta}_j^{ini} }[/math] is an initial estimate of the coefficients obtained through ridge regression. Adaptive Lasso ends up penalizing more those coefficients with lower initial estimates. [math]\displaystyle{ \gamma }[/math] is a positive constant for adjustment of the adaptive weight vector, and the authors suggest the possible values of 0.5, 1 and 2.
  • When n goes to infinity, [math]\displaystyle{ \hat{\omega}_j |\beta_j| }[/math] converges to [math]\displaystyle{ I(\beta_j \neq 0) }[/math]. So the adaptive Lasso procedure can be regarded as an automatic implementation of best-subset selection in some asymptotic sense.
  • What is the oracle property of an estimator? An oracle estimator must be consistent in 1) variable selection and 2) consistent parameter estimation.
  • Oracle property: Oracle property is a name given to techniques for estimating the regression parameters in the models fitted to high-dimensional data which have the property that they can correctly select the nonzero coefficients with the probability converging to one and that the estimators of nonzero coefficients are asymptotically normal with the identical means and covariances that they would have if the zero coefficients were known in advance that is the estimators are asymptotically as efficient as the ideal estimation assisted by an 'oracle' who knows which coefficients are nonzero.
  • (Linear regression) The adaptive lasso and its oracle properties Zou (2006, JASA)
  • (Cox model) Adaptive-LASSO for Cox's proportional hazard model by Zhang and Lu (2007, Biometrika)
  • When the LASSO fails???. Adaptive lasso is used to demonstrate its usefulness.

Cyclic coordinate descent

A deep dive into glmnet: type.gaussian

Survival data

data(CoxExample)
dim(x) # 1000 x 30
ind.train <- 1:nrow(x)/2
cv.fit <- cv.glmnet(x[ind.train, ], y[ind.train, ], family="cox")
coef(cv.fit, s = "lambda.min") 
    # 30 x 1, coef(...) is equivalent to predict(type="coefficients",...)
pr <- predict(cv.fit, x[-ind.train, ], type = "link", s = "lambda.min")
    # the default option for type is 'link' which gives the linear predictors for "cox" models. 
pr2 <- x[-ind.train, ] %*% coef(cv.fit, s = "lambda.min")
all.equal(pr[, 1], pr2[, 1]) # [1] TRUE
class(pr) # [1] "matrix"
class(pr2) # [1] "dgeMatrix"
# we can also use predict() with glmnet object
pr22 <- predict(fit, x[-ind.train, ], type='link', s = cv.fit$lambda.min)
all.equal(pr[, 1], pr22[, 1]) # [1] TRUE

pr3 <- predict(cv.fit, x[-ind.train, ], type = "response", s = "lambda.min")
range(pr3)  # relative risk [1]  0.05310623 19.80143519

glmnet 4.0: family

glmnet v4.0: generalizing the family parameter

Timing

nvec <- c(100, 400)
pvec <- c(1000, 5000)
for(n in nvec)
  for(p in pvec) {
    nzc = trunc(p/10)  # 1/10 variables are non-zeros 
    x = matrix(rnorm(n * p), n, p)
    beta = rnorm(nzc)
    fx = x[, seq(nzc)] %*% beta/3
    hx = exp(fx)
    ty = rexp(n, hx)
    tcens = rbinom(n = n, prob = 0.3, size = 1)  # censoring indicator
    y = cbind(time = ty, status = 1 - tcens)  # y=Surv(ty,1-tcens) with library(survival)
    foldid = sample(rep(seq(10), length = n))
    o <- system.time(fit1_cv <- cv.glmnet(x, y, family = "cox", foldid = foldid) )
    cat(sprintf("n=%1d, p=%5d, time=%8.3f\n", n, p, o['elapsed']))
}

Running on core i7 2.6GHz, macbook pro 2018. Unit is seconds.

p ∖ n 100 400
1000 5 58
5000 9 96

Xeon E5-2680 v4 @ 2.40GHz

p ∖ n 100 400
1000 7 98
5000 13 125

warm start

caret

coefficients from glmnet and caret are different for the same lambda

All interactions

How to make all interactions before using glmnet

Discussion

Prediction

ROC

How to plot ROC-curve for logistic regression (LASSO) in R?

LASSO vs Least angle regression

Tidymodel

Lasso regression using tidymodels and #tidytuesday data for the office

Binary particle swarm optimization (BPSO)

An efficient gene selection method for microarray data based on LASSO and BPSO

Retrieved from "https://wiki.taichimd.us/index.php?title=Glmnet&oldid=25336"